| THE MUSCLES 75 are connected with the bones, cartilages, ligaments, and skin, either directly, or through the intervention of fibrous structures called tendons or aponeuroses. Where a muscle is attached to bone or cartilage, the fibers end in blunt extremities upon the periosteum or perichondrium, and do not come into direct relation with the osseous or cartilaginous tissue. Where muscles are connected with its skin, they lie as a flattened layer beneath it, and are connected with its areolar tissue by larger or smaller bundles of fibers, as in the muscles of the face. | 1 |
| The muscles vary extremely in their form. In the limbs, they are of considerable length, especially the more superficial ones; they surround the bones, and constitute an important protection to the various joints. In the trunk, they are broad, flattened, and expanded, and assist in forming the walls of the trunk cavities. Hence the reason of the terms, long, broad, short, etc., used in the description of a muscle. | 2 |
| There is considerable variation in the arrangement of the fibers of certain muscles with reference to the tendons to which they are attached. In some muscles the fibers are parallel and run directly from their origin to their insertion; these are quadrilateral muscles, such as the Thyreohyoideus. A modification of these is found in the fusiform muscles, in which the fibers are not quite parallel, but slightly curved, so that the muscle tapers at either end; in their actions, however, they resemble the quadrilateral muscles. Secondly, in other muscles the fibers are convergent; arising by a broad origin, they converge to a narrow or pointed insertion. This arrangement of fibers is found in the triangular muscles—e. g., the Temporalis. In some muscles, which otherwise would belong to the quadrilateral or triangular type, the origin and insertion are not in the same plane, but the plane of the line of origin intersects that of the line of insertion; such is the case in the Pectineus. Thirdly, in some muscles (e. g., the Peronei) the fibers are oblique and converge, like the plumes of a quill pen, to one side of a tendon which runs the entire length of the muscle; such muscles are termed unipennate. A modification of this condition is found where oblique fibers converge to both sides of a central tendon; these are called bipennate, and an example is afforded in the Rectus femoris. Finally, there are muscles in which the fibers are arranged in curved bundles in one or more planes, as in the Sphincters. The arrangement of the fibers is of considerable importance in respect to the relative strength and range of movement of the muscle. Those muscles where the fibers are long and few in number have great range, but diminished strength; where, on the other hand, the fibers are short and more numerous, there is great power, but lessened range. | 3 |
| The names applied to the various muscles have been derived: (1) from their situation, as the Tibialis, Radialis, Ulnaris, Peronæus; (2) from their direction, as the Rectus abdominis, Obliqui capitis, Transversus abdominis; (3) from their uses, as Flexors, Extensors, Abductors, etc.; (4) from their shape, as the Deltoideus, Rhomboideus; (5) from the number of their divisions, as the Biceps and Triceps; (6) from their points of attachment, as the Sternocleidomastoideus, Sternohyoideus, Sternothyreoideus. | 4 |
| In the description of a muscle, the term origin is meant to imply its more fixed or central attachment; and the term insertion the movable point on which the force of the muscle is applied; but the origin is absolutely fixed in only a small number of muscles, such as those of the face which are attached by one extremity to immovable bones, and by the other to the movable integument; in the greater number, the muscle can be made to act from either extremity. | 5 |
| In the dissection of the muscles, attention should be directed to the exact origin, insertion, and actions of each, and to its more important relations with surrounding parts. While accurate knowledge of the points of attachment of the muscles is of great importance in the determination of their actions, it is not to be regarded as conclusive. The action of the muscle deduced from its attachments, or even by pulling on it in the dead subject, is not necessarily its action in the living. By pulling, for example, on the Brachioradialis in the cadaver the hand may be slightly supinated when in the prone position and slightly pronated when in the supine position, but there is no evidence that these actions are performed by the muscle during life. It is impossible for an individual to throw into action any one muscle; in other words, movements, not muscles, are represented in the central nervous system. To carry out a movement a definite combination of muscles is called into play, and the individual has no power either to leave out a muscle from this combination or to add one to it. One (or more) muscle of the combination is the chief moving force; when this muscle passes over more than one joint other muscles (synergic muscles) come into play to inhibit the movements not required; a third set of muscles (fixation muscles) fix the limb—i. e., in the case of the limb-movements—and also prevent disturbances of the equilibrium of the body generally. As an example, the movement of the closing of the fist may be considered: (1) the prime movers are the Flexores digitorum, Flexor pollicis longus, and the small muscles of the thumb; (2) the synergic muscles are the Extensores carpi, which prevent flexion of the wrist; while (3) the fixation muscles are the Biceps and Triceps brachii, which steady the elbow and shoulder. A further point which must be borne in mind in considering the actions of muscles is that in certain positions a movement can be effected by gravity, and in such a case the muscles acting are the antagonists of those which might be supposed to be in action. Thus in flexing the trunk when no resistance is interposed the Sacrospinales contract to regulate the action of gravity, and the Recti abdominis are relaxed. 76 | 6 |
| By a consideration of the action of the muscles, the surgeon is able to explain the causes of displacement in various forms of fracture, and the causes which produce distortion in various deformities, and, consequently, to adopt appropriate treatment in each case. The relations, also, of some of the muscles, especially those in immediate apposition with the larger bloodvessels, and the surface markings they produce, should be remembered, as they form useful guides in the application of ligatures to those vessels. | 7 |
| |
| 1. Mechanics of Muscle |
| |
| In studying the mechanical action of muscles 77 the individual muscle cannot always be treated as a single unit, since different parts of the same muscle may have entirely different actions, as with the Pectoralis major, the Deltoid, and the Trapezius where the nerve impulses control and stimulate different portions of the muscle in succession or at different times. Most muscles are, however, in a mechanical sense units. But in either case the muscle fibers constitute the elementary motor elements. | 8 |
 |
FIG. 361– No caption. (See enlarged image) |
| |
| |
| The Direction of the Muscle Pull.—In those muscles where the fibers always run in a straight line from origin to insertion in all positions of the joint, a straight line joining the middle of the surface of origin with the middle of the insertion surface will give the direction of the pull (Fig. 361). If, however, the muscle or its tendon is bent out of a straight line by a bony process or ligament so that it runs over a pulley-like arrangement, the direction of the muscle pull is naturally bent out of line. The direction of the pull in such cases is from the middle point of insertion to the middle point of the pulley where the muscle or tendon is bent. Muscles or tendons of muscles which pass over more than one joint and pass through more than one pulley may be resolved, so far as the direction of the pull is concerned, into two or more units or single-joint muscles (Fig. 362). The tendons of the Flexor profundus digitorum, for example, pass through several pulleys formed by fibrous sheaths. The direction of the pull is different for each joint and varies for each joint according to the position of the bones. The direction is determined in each case, however, by a straight line between the centers of the pulleys on either side of the joint (Fig. 363). The direction of the pull in any of the segments would not be altered by any change in the position or origin of the muscle belly above the proximal pulley. | 9 |
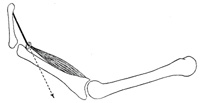 |
FIG. 362– No caption. (See enlarged image) |
| |
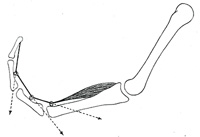 |
FIG. 363– No caption. (See enlarged image) |
| |
| |
| The Action of the Muscle Pull on the Tendon.—Where the muscle fibers are parallel or nearly parallel to the direction of the tendon the entire strength of the muscle contraction acts in the direction of the tendon. | 10 |
| In pinnate muscles, however, only a portion of the strength of contraction is efficient in the direction of the tendon, since a portion of the pull would tend to draw the tendon to one side, this is mostly annulled by pressure of surrounding parts. In bipinnate muscles this lateral pull is counterbalanced. If, for example, the muscle fibers are inserted into the tendon at an angle of 60 degrees (Fig. 364), it is easy to determine by the parallelogram of forces that the strength of the pull along the direction of the tendon is equal to one-half the muscle pull. | 11 |
| T = tendon, m = strength and direction of muscle pull. | 12 |
| t = component acting in the direction of the tendon. | 13 |
| φ = angle of insertion of muscle fibers into tendon. | 14 |
| cos φ = t/m cos ∠ 60° = 0.50000 | 15 |
| 0.5 = t/m t = 1/2 m | 16 |
| If |
< φ = 72° 30' |
cos = 1/3 |
| < φ = 41° 20' |
cos = 3/4 |
| < φ = 90° |
cos = 0 |
| < φ = 0° |
cos = 1 |
|
| The more acute the angle φ, that is the smaller the angle, the greater the component acting in the direction of the tendon pull. At 41° 20’ three-fourths of the pull would be exerted in the direction of the tendon and at 0° the entire strength. On the other hand, the greater the angle the smaller the tendon component; at 72° 30’ one-third the muscle strength would act in the direction of the tendon and at 90° the tendon component would be nil. | 17 |
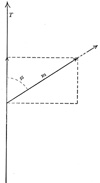 |
FIG. 364– No caption. (See enlarged image) |
| |
| |
| The Strength of Muscles.—The strength of a muscle depends upon the number of fibers in what is known as the physiological cross-section, that is, a section which passes through practically all of the fibers. In a muscle with parallel or nearly parallel fibers which have the same direction as the tendon this corresponds to the anatomical cross-section, but in unipinnate and bipinnate muscles the physiological cross-section may be nearly at right angles to the anatomical cross-section as shown in Fig. 365. Since Huber has shown that muscle fibers in a single fasciculus of a given muscle vary greatly in length, in some fasciculi from 9 mm. to 30.4 mm., it is unlikely that the physiological cross-section will pass through all the fibers. Estimates have been made of the strength of muscles and it is probable that coarse-fibered muscles are somewhat stronger per square centimeter of physiological cross-section than are the fine-fibered muscles. Fick estimates the average strength as about 10 kg. per square cm. This is known as the absolute muscle strength. The total strength of a muscle would be equal to the number of square centimeters in its physiological cross-section x 10 kg. | 18 |
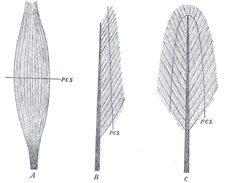 |
FIG. 365– A, fusiform; B, unipinnate; C, bipinnate; P.C.S., physiological cross-section. (See enlarged image) |
| |
| |
| The work Accomplished by Muscles.—For practical uses this should be expressed in kilogrammeters. In order to reckon the amount of work which a muscle can perform under the most favorable conditions it is necessary to know (1) its physiological cross-section (2) the maximum shortening, and (3) the position of the joint when the latter is obtained. | 19 |
| Work = lifted weight x height through which the weight is lifted; or | 20 |
| Work = tension x distance; tension = physiological cross-section x absolute muscle strength. | 21 |
| If a muscle has a physiological cross-section of 5 sq. cm. its tension strength = 5 x 10 or 50 kg. If it shortens 5 cm. the work = 50 x .05 = 2.5 kilogrammeters. If one determines then the physiological cross-section and multiplies the absolute muscle strength, 10 kg. by this, the amount of tension is easily obtained. Then one must determine only the amount of shortening of the muscle for any particular position of the joint in order to determine the amount of work the muscle can do, since work = tension x distance. | 22 |
| The tension of a muscle is, however, not constant during the course of contraction but is continually decreasing during contraction. It is at a maximum at the beginning and gradually decreases. | 23 |
This can be illustrated by the work diagram Fig. 366.
| A M D (ordinate) = tension. |
| A V X (abscissa) = shortening. |
| A D = tension of muscle in extended or antagonistic position. |
| A V = amount of actual shortening. |
| A M = tension in midposition = absolute muscle strength. |
| D V = shows how the tension sinks from maximum (in the extended position of the muscle) where it is about double that in the midposition (M) to nothing on complete contraction. |
| Δ A D V = work diagram, in reality the hypothenose is not straight but has a concave curve. The Δ has the same area as the rectangle A M M’ V. |
| A M = the average tension. |
| Work = A M x A V kilogrammeters if the size of the ordinate as expressed in kilograms and the abscissa in meters. |
| 24 |
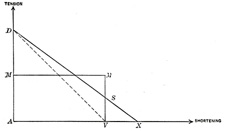 |
FIG. 366– No caption. (See enlarged image) |
| |
| Although the muscle works with a changing tension, yet the accomplishment is the same as if it were contracting with the tension of the midposition. | 25 |
| In reality the amount of work is somewhat greater since even in extreme contraction the muscle still retains a certain amount of tension so that the maximum amount of work is more nearly like A D X. We know that a muscle may have an extreme actual shortening of about 80 per cent. of its length when the tendon of insertion is cut. | 26 |
| The trapezoid A D S V represents more nearly the amount of work, but since there are only approximate values and A D S V is not much larger than A M M’ V, we may use the latter. | 27 |
| Only the tension and amount of shortening are needed to determine the amount of work of the muscle. Neither the lever arm nor the fiber angle in pinnate muscles need be considered. | 28 |
| The diagram Fig. 367 shows that the lever arm is of no importance for determining the amount of work the muscle performs. | 29 |
| J B and J B1 = two bones jointed at J. C D and E F = the direction of the pull of two muscles of equal cross-section, each having a muscle tension of 1000 gms. | 30 |
| The centers of the attachments are such that perpendiculars J c and J e to C D and E F are equal to 40 and 23 mm. respectively, J c = 40 mm. and J e = 23 mm. The static moments are equal to 1000 x 40 and 1000 x 23, therefore the first muscle can hold a much larger load (L) on the bone J B1 at H1 (100 mm. from J) than the second muscle whose load can be designated as L1. | 31 |
Equilibrium exists for the first muscle if
L x 100 = 1000 x 40 or L = 1000 x 40/100 = 400 gms. | 32 |
For the second muscle L1 x 100 = 1000 x 23.
L1 = 1000 x 23/100 = 230 gms. | 33 |
| If we suppose J B to be fixed and J B1 to move in the plane of the paper about J and the muscle C D to shorten 5 mm. C d = C D − 5 mm. and with the tension of 1000 gms., J B1 will take the position J B2 and the load (L) will be lifted from H1 to H2. | 34 |
| If the second muscle likewise shortens 5 mm. then E f = E F — 5 mm., and with the tension of 1000 gms. the bone J B1 will take the position J B3 and the weight or load (L1) will be lifted from H1 to H3. The question now is to prove that the work done is the same in both cases, namely, 5 x 1000 grammillimeters. If so, 400 x H1 H2 = 230 x H1 H3 = 5000 grammillimeters. | 35 |
| Since the two radii C d and C d’ are very long as compared with the arc d d’ we may consider this short arc as a line \??\ to C D at d’, likewise the arc f f’ may be considered as a straight line \??\ to E F. In the same manner we can consider the short arcs F f, D d, H1 H2 and H1 H3 \??\ to the line J B1. The sides D d’ and F f’ of the Δ D d d’ and F f f’ are each 5 mm. | 36 |
| The lever arm D J = 60 mm. and J F = 30 mm. | 37 |
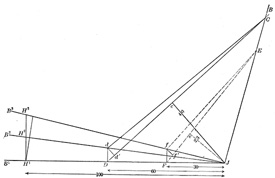 |
FIG. 367– No caption. (See enlarged image) |
| |
| The Δ D d d' is similar to the Δ D c J | 38 |
| hence D d |
: 5 :: 60 : 40 |
D d = 300/40 |
| also H1 H2 |
: D d :: 100 : 60 |
| H1 H2 |
: 300/40 :: 100 : 60 |
H1 H2 = 300/24 |
|
| hence F f |
: 5 :: 30 : 23 |
F f = 150/23 |
| also H1 H3 |
: F f :: 100 : 30 |
| H1 H3 |
:150/23::100:30 |
H1 H3 = 1500/69 |
| … 400 x 300/24 = 230 x 1500/69 = 5000 |
|
| Thus we see that the work of the two muscles depends on the size of the contraction and on the tension and not on the lever arm in very small contractions or in the summation of such contractions and therefore for large contractions. In the first muscle a large load is moved through a short distance and in the second muscle a lighter load is moved through a greater distance. | 39 |
| The amount of work accomplished by pinnate muscles is not dependent upon the angle of insertion of the muscle fibers into the tendon, as will be seen by the following diagram Fig. 368. | 40 |
| T' T = | direction of the tendon pull. |
| w a = | direction of muscle fiber before contraction. |
| m’ = | direction of muscle fiber after contraction. |
| v = | amount of contraction. |
| m = | tension of the muscle. |
| φ = | angle of insertion of muscle fiber. |
| t = | tendon component = m x cos φ = the weight carried by the tendon to balance the muscle tension. |
| d = | distance tendon is drawn up. |
| (1) m x v = | work done by the muscle fiber. |
| (2) t x d = | work done by the movement of the tendon. |
|
| If we consider the distance v as being very short then the line b c can be dealt with as though it were perpendicular to a c. | 41 |
| then v = d x cos φ or d = v/cos φ
since t = m x cos φ or m = t/cos φ
m x v = t/cos φ x d x cos φ = t x d | 42 |
| If this is true for very minute contractions it is likewise true for a series of such contraction and hence for larger contractions. | 43 |
| If we assume that φ = 60°, m = 10 kg. and v = 5 mm., the work done by the contracting muscle fiber = m v or 10 x 5 kilogrammillimeters. | 44 |
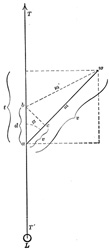 |
FIG. 368– No caption. (See enlarged image) |
| |
cos ∠ 60° = 1/2; hence t = 1/2 m; and d = v/1/2 = 2 v; 1/2 m = 5 kg.; and 2 v = 10 mm. hence t d = 50 kilogrammillimeters or the work done by the movement of the tendon in lifting the load of 5 kg. a distance of 10 mm., and is exactly the same as that done by the muscle fiber. The load on the tendon is but one-half the tension of the muscle, but the distance through which the load is lifted is twice that of the amount of shortening of the muscle.
If φ = 41° 20’ then cos φ = 3/4
hence t = 3/4 m and d = 4/3 v and t d = m v | 45 |
| In pinnate muscles, then, we have the rather unexpected condition in which the same amount of movement of the tendon can be accomplished with less contraction of the muscle than in muscles where the fibers have the same direction as the tendon. | 46 |
| |
| The Action of Muscles on Joints.—If we consider now the action of a single muscle extending over a single joint in which one bone is fixed and the other movable, we will find that muscle pull can be resolved into two components, a turning component and a friction or pressure component as shown in Fig. 369. | 47 |
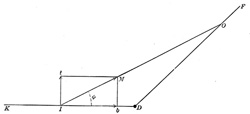 |
FIG. 369– No caption. (See enlarged image) |
| |
| D F = the fixed bone from which the muscle takes its origin. | 48 |
| D K = the movable bone. | 49 |
| O I = a line from the middle of origin to the middle of insertion. | 50 |
| I M = size and direction of the muscle pull. | 51 |
| If the parallelogram is constructed with I t and M b ⊥ to D K, then I t = the turning component and I b = the component which acts against the joint. | 52 |
| The size of the two components depends upon the insertion angle φ. The smaller this angle the smaller the turning component, and the nearer this angle φ is to 90° the larger the turning component. | 53 |
| I t = I M x sin φ | 54 |
| I b = I M x cos φ | 55 |
| If φ = 90° cos φ = 0, sine φ = 1 hence I b = 0 and I t = I m | 56 |
| If φ = 0° cos φ = 1, sine φ = 0 hence I b = 1 and I t = 0 | 57 |
| With movements of the bone D K the angle of insertion is continually changing, and hence the two components are changing in value. | 58 |
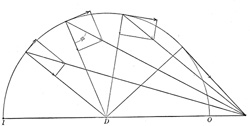 |
FIG. 370– No caption. (See enlarged image) |
| |
| If, for example, the distance from origin 0 to the joint D is greater than from D to I, as in the Brachialis or Biceps muscles, the turning component increases until the insertion angle φ = 90°, which is the optimum angle for muscle action, while the pressure component gradually decreases. If the movement continues beyond this point the turning component gradually decreases and the pressure component changes into a component which tends to draw the two bones apart and which gradually increases as shown in Fig. 370. | 59 |
| When the bone D K is in such a position that the insertion angle φ = 41° 20’ the pressure component = 3/4 I m and the turning component 1/4 I m, at 60° the two components are equal, at 90° the pressure component = 0 and the turning component = I M and at 131° 21’ the pressure component has been converted into a pulling component = 1/4 I M and the turning component = 3/4 I M. | 60 |
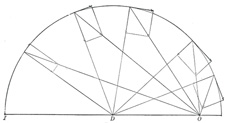 |
FIG. 371– No caption. (See enlarged image) |
| |
| If, for example, the distance from the origin O to the joint D is less than the distance from the insertion I to the joint D, as in the Brachioradialis muscle, the insertion angle increases with the flexion but never reaches 90°. The turning component gradually increases to a certain point and then slowly decreases as shown in Fig. 371, while the pressure component gradually decreases and then slowly increases. It always remains large and its action is always in the direction of the joint. | 61 |
| |
| Levers.—The majority of the muscles of the body act on bones as the power on levers. Levers of the III class are the most common, as the action of the Biceps, and the Brachialis muscles on the forearm bones. Levers of the I Class are found in movements of the head where the occipito-atlantal joint acts as the fulcrum and the muscles on the back of the neck as the power. Another common example is | 62 |
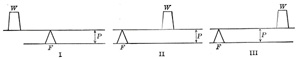 |
FIG. 372– No caption. (See enlarged image) |
| |
| the foot when one raises the body by contracting the Gastrocnemius and Soleus. Here the ankle-joint acts as the fulcrum and the pressure of the toes on the ground as the weight. This is frequently, though wrongly, considered a lever of the II Class. If one were to stand on one’s head with the legs up and with a weight on the plantar surface of the toes, it is easy to see that we would have a lever of the I Class if the weight were raised by contraction of the Gastrocnemius muscle. The confusion has arisen by not considering the fact that the fulcrum and the power in all three classes of levers must have a common basis of action, as shown in Fig. 372. | 63 |
| If the fulcrum rests on the earth the power must either directly or indirectly push from the earth or be attached to the earth either by gravity or otherwise if it pulls toward the earth. If the power were attached to the weight no lever action could be obtained. | 64 |
| There are no levers of the II Class represented in the body. | 65 |











